Applications of pigeonhole principle with example. Applications Of The Pigeonhole Principle Mathematics Essay 2023-01-02
Applications of pigeonhole principle with example
Rating:
6,2/10
859
reviews
The pigeonhole principle, also known as the "boxes and pigeons" principle, is a simple but powerful concept in mathematics that states that if there are more objects than available spaces (or "pigeonholes"), then at least one space must contain more than one object. This principle has many applications in various fields, including computer science, economics, and even daily life.
One of the most common applications of the pigeonhole principle is in computer science, specifically in the field of data compression. In data compression, the goal is to represent a large amount of data using a smaller number of bits. One way to do this is by using a technique called "lossless compression," where the original data can be recovered exactly from the compressed version. The pigeonhole principle can be used to prove that certain lossless compression schemes are optimal, meaning that no other scheme can compress the data more efficiently. For example, suppose we have a set of data consisting of the letters A, B, C, and D. If we want to represent this data using only 2 bits per letter, we can use the pigeonhole principle to prove that at least one of the letters must be represented by two different combinations of 2 bits. This means that the data cannot be losslessly compressed using 2 bits per letter, and we must use a different method or a higher number of bits to achieve optimal compression.
Another application of the pigeonhole principle is in economics, specifically in the study of market equilibrium. Market equilibrium occurs when the quantity of a good or service that is being supplied is equal to the quantity that is being demanded. The pigeonhole principle can be used to prove that under certain conditions, market equilibrium is always possible. For example, suppose we have a market for a certain type of good, and there are three sellers who each have a certain number of units of the good to sell. The pigeonhole principle states that if the sellers have a total of more than three units of the good, then at least one of them must have more than one unit to sell. This means that there must be at least one buyer who is willing to purchase more than one unit of the good, which is necessary for the market to reach equilibrium.
In daily life, the pigeonhole principle can also be used to solve practical problems. For example, suppose you have a group of friends who are going on a road trip, and you need to decide which car to take. You have three cars to choose from, each with a different number of seats. The pigeonhole principle states that if you have more friends than the total number of seats in the three cars, then at least one of the cars must have more than one person in it. This can help you decide which car to take, and also serve as a reminder to carpool to save space and reduce environmental impact.
In conclusion, the pigeonhole principle is a simple but powerful concept that has many applications in various fields, including computer science, economics, and daily life. Its versatility and simplicity make it a valuable tool for solving a wide range of problems.
(PDF) Pigeonhole Principle: Real Life Applications and Mathematical Investigation

Thus, we are right to say that there are at least 2 people who have their birthday falling in the same month. ABSTRACT Given two graphs GG and HH, let f G,H f G,H denote the maximum number cc for which there is a way to color the edges of GG with cc colors such that every subgraph HH of GG has at least two edges of the same color. Suppose that there are 50 people in the room. Proof: Let us label the n pigeonholes 1, 2, …, n, and the m pigeons p 1, p 2, …, p m. Permutation Card Trick 158. Suppose that a flock of 20 pigeons flies into a set of 19 pigeonholes to roost.
Next
Art of Problem Solving

What about the socks? Although this principle may seem obvious to some, many challenging Olympiad problems can be solved by applying it. For example, the nonexistence of a universal lossless compression algorithm an algorithm that always compresses a string of characters into a shorter string of characters can be shown to be impossible by using a pigeonhole argument that shows that two strings would have to be compressed into the same compressed representation, making it impossible to losslessly decompress the string. While this version sounds different, it is mathematically the same as the one stated with pigeons and pigeonholes. In simpler terms, there exists some value of more than one pigeon per hole. The same proof can be replicated for the pairwise sums, but the differences give a better constant.
Next
proofs

To prove the result, we may divide the square into 25 equal smaller squares of side units each. Combinatorial Card Trick : Heres the trick: A magician asks an unsuspecting observer to randomly choose five cards from a standard deck of playing cards. Case 2: someone has no friends If someone lacks any friends, then that person is a stranger to all other guests. Seem to me that it would be possible to select 13 cards all of the same suit and that 14 would be the answer to 7 pigeonhole principle. Pigeonhole Principle and divisibility Consider the following random list of 12 numbers say, 2, 4, 6, 8, 11, 15, 23, 34, 55, 67, 78 and 83.
Next
soft question

Problem 1 If a man has an infinite number of four different patterns of multicolored socks in a drawer, how many socks must he pull out of the drawer to guarantee he has a pair? This generalized version may sound different, but it is mathematically the same as the one explained using pigeons and pigeonholes. Case 2: the point is not connected to at least three other points If any of these points are not connected to each other, then we have found a triangle of three mutual strangers. A proof due to Seidenberg uses the pigeonhole principle. How does the trick work? This means the maximum value should also be larger than one. Some of them are acquainted with each other, while some not. The first card that the accomplice shows to the magician is one of these two cards.
Next
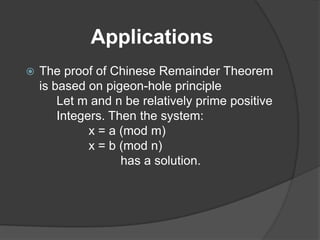
Applications of Pigeonhole Principle

Thus we have mn+1 ordered pairs, of which at most mn are distinct. Example 08 20 cards, numbered from 1 to 20, are placed face down on a table. To prove that the final statement is always true, we first divide the circle into six equal sectors as shown; Allowing each sector to be a pigeonhole and each dart to be a pigeon, we have seven pigeons to go into six pigeonholes. The maximum must at least be the average, so there must be a birthday that at least 820 people share. The Pigeonhole Principle sounds trivial but its uses are deceiving astonishing! Question: I would like to work on this amazing principle with my students for a week and was, therefore, gathering problems related to the Pigeonhole Principle with beautiful solutions.
Next
Applications Of The Pigeonhole Principle Mathematics Essay
Graduate level applications would be fine as well, but I am mostly interested in examples that I can use in my undergrad classes. Without loss of generality, we may assume that a precedes b in the sequence. If he decides to use the increasing subsequence, he should leave the first four cards untouched and flips the other six cards over in a leftward manner as shown: 1 7 4 2 6 9 Direction of flip The magician on realising that the four missing numbers are 3, 5, 8 and 10 and the leftward direction of flip, will thus proclaim the 4 hidden numbers to be 3, 5, 8, and 10 respectively! Please note, I am the author of the blog. What are the pigeons and what are the holes? We will argue that when n people meet and some subset of them shake hands, at least 2 of them must have shaken hands with exactly the same number of other people. In such a case, the circle is this time divided into five sectors and all else follows. Imagine the six people as points and let an edge between points indicate friendship. I n New York City, there are two non-bald people who have the same number of hairs on their head.
Next
CC

If you like the posts and videos, please consider a pledge on I post to the following sites, mostly with updates for new content. However, take note that this is not always true anymore with only five darts or less. For instance, 13 people are involved in a survey to determine the month of their birthday. In our example, the 6 of Clubs will be ranked 1, the 5 of Spades will be ranked 2 and the 2 of Diamonds will be ranked 3. The first card that the accomplice shows to the magician is one of these two cards.
Next
Discrete Mathematics
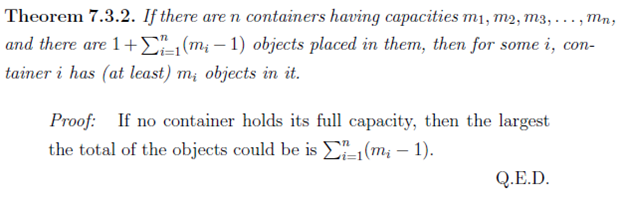
Therefore everyone has between 0 to n— 2 friends. So nice example, but not really practical I think. For each row of 4 points, in reference to the pigeon hole method, two must be the same color, for instance, green. How do we know for sure without having to ask every single person? Use MathJax to format equations. For each number x in the sequence, we have the ordered pair i, j , where i is the length of the longest increasing subsequence beginning with x, and j is the length of the longest decreasing subsequence ending with x. Can you see why we are now done? The magician now knows that the suit of the mystery card is hearts.
Next






