Equation reducible to quadratic equation. Equations Reducible to Quadratic form 2022-12-30

Equation reducible to quadratic equation Rating: 8,6/10 148 reviews

An equation that can be reduced to a quadratic equation is one that can be rewritten in the form of ax^2 + bx + c = 0, where a, b, and c are constants and x is the variable. Quadratic equations have the unique property that they can be solved using the quadratic formula, which allows us to find the values of x that make the equation true.

There are many types of equations that can be reduced to quadratic form. One common example is a polynomial equation of degree 2 or higher. For instance, the equation x^3 + 2x^2 + 3x + 4 = 0 can be rewritten as x^2 + 2x + (3/x) + 4/x^2 = 0, which is a quadratic equation. By using the quadratic formula, we can solve for the values of x that make this equation true.

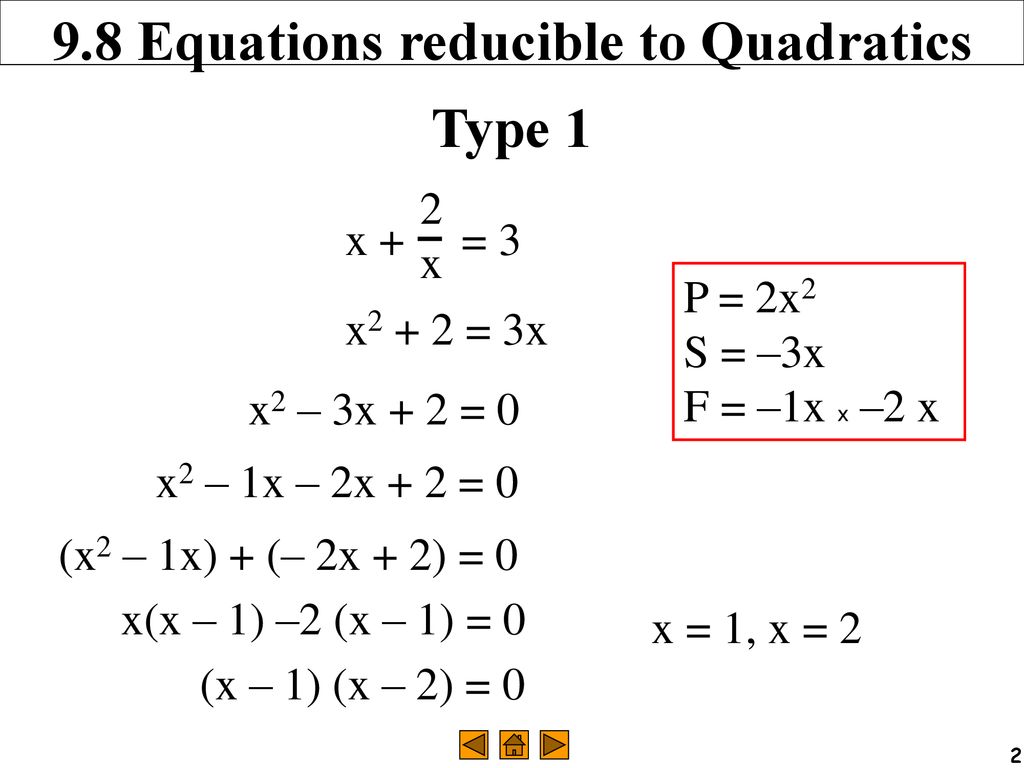
Another type of equation that can be reduced to quadratic form is a rational equation. This is an equation that includes terms with variables in the denominator, such as (2x + 3)/(x^2 + 4x + 5) = 0. By multiplying both sides of the equation by the denominator and simplifying, we can rewrite this equation as a quadratic equation and solve it using the quadratic formula.

In addition to polynomial and rational equations, there are other types of equations that can be reduced to quadratic form as well. For example, an exponential equation of the form a^x = b can be rewritten as x = log_a(b), which is a quadratic equation if we let x = log_a(b) and solve for a. Similarly, a logarithmic equation of the form log_a(x) = b can be rewritten as a^b = x, which is a quadratic equation if we let a^b = x and solve for a.

Reducing an equation to quadratic form can be a useful technique when trying to solve for the values of a variable. While the quadratic formula can only be used to solve quadratic equations, many other types of equations can be rewritten in quadratic form and then solved using this formula. This allows us to find solutions to a wide range of mathematical problems, from simple algebraic equations to more complex equations involving higher-order polynomials, rational expressions, and other types of functions.

Equations Reducible to Quadratic Form

equation reducible to quadratic equation

Explanation: can be rewritten in quadratic form by setting , and, consequently, ; the resulting equation is as follows: By the reverse-FOIL method we can factor the trinomial at left. The exceptions will be seen in the examples below. Conclusion This assignment describes the root quadratic equation and the useful circumstances of the reducible amount in mathematics. What Are Quadratic Equations? A: Some of the equations of various types can be reduced to quadratic form. A quadratic equation that can be solved by any method and back substitute the value to find the actual roots of the equation.


Next

Equations Reducible to Quadratic Form (Part 1)

equation reducible to quadratic equation

Also, the complex root, factorisation, graphing, and finding the root is generally preferred by the mathematician for the quadratic value. Example 1 Solve for x: 9 x — 4. Substituting for , we get , in which case , or in which case. We are converting the equation to a quadratic by making the substitution. Ways to Solve Quadratic Equation to Reducible Different primary methods are applicable to check if an equation is reducible or not.

Next

Tips to Solve Equations reducible to Quadratic

equation reducible to quadratic equation

The example below explains the method. By the term reducible, it seems to include the quadratic formula to convert in the substitution. The quadratic equation will have two roots. These equations can be easily solved if we convert them to a quadratic form which is otherwise difficult to solve. Do you know how to proceed here? We are looking for two integers with sum 8 and product 12; they are 2 and 6, so the equation becomes Setting both binomials equal to 0, it follows that or. What Is Referred To By Discriminant? This lesson will discuss equations that can be reduced or converted to quadratic equations, using simple substitutions. Is Quadratic a Reducible? Then we follow any convenient method to factorise the equation, either by using quadratic formula or splitting the middle term method.

Next

Online Tutoring

equation reducible to quadratic equation

Using the laws of exponents, these can be respectively expressed as 2 3. For getting the appropriate value in the equation the best part is used for this is making a reducible value by applying the substitution in the formula. We were supposed to find the value of x. The factorisation is referred to as the most possible case and one of the best scenarios for using the quadratic formula in maths. Method: In this type of equation, we square the given equation on both sides to obtain the equation in quadratic form.

Next

Fraction Equations Reducible to Quadratic

equation reducible to quadratic equation

From these two equations, we get four solutions: 1, —1, 3, and —3. Some forms are biquadratic equations, reciprocal equations, and irrational equations reducible to quadratics. They are advanced to linear functions and give a significant move away from attachment to straight lines. Some real-life applications are calculating the area to determine the products, profit or formulating the speed of a vehicle. A: Quadratic functions are essential and unique part of the school curriculum.

Next

Learn Equations Reducible to Quadratic Equations With Examples

equation reducible to quadratic equation

A: Quadratic equations are equations with at least one squared variable. In the basics of mathematics, the substitution can be taken as y replacing x 2 for containing the formula successfully applying. Now by applying the factor theorem to check the possible values by trial and error. The cubic equation will have three roots, like a quadratic equation with two real roots. . Roots of the quadratic equation can be referred to as the most useful and simple formula to get the reducible value. Proof of Quadratic Equation Formula of Reducible In the reducible formula, there are different use cases of the quadratic equation in applying the value of x 2 to generate the actual amount that is desirable as the reducible amount.

Next

Algebra

equation reducible to quadratic equation

Factorization of Quadratic Formula to Make the Value Reducible Converting the linear factors of the equation is more convertible for expressing the method of the quadratic formula by applying the equation. The factorisation can engage the state of the formula in converting a complex root. The motto of applying factorisation can be included in the development of the factor that is stated equal to zero. We are looking for two integers with sum 8 and product 12; they are and , so the equation becomes Setting both binomials equal to 0, it follows that or. Equations Reducible to Quadratic Equations: In this article, we will discuss the equations that are not quadratic but are reducible to quadratic equations. In most cases, check the exponents of the given equation.

Next

Equations Reducible to Quadratic form

equation reducible to quadratic equation

We solve a cubic equation by reducing it to a quadratic equation. Let us learn the different types of equations that can be reduced to quadratic form with solved examples. Dividing the equation by both sides in the preferable application of applying square by both sides can make the square complete. We can formulate the quadratic equation for the given data in real-life examples and solve them to find the unknown value. And these were the solutions we were looking for. One of the ways to begin is factorization. The general application of algebra in mathematics may include the use case of the quadratic formula as the proof of completing the square.

Next

5. Equations Reducible to Quadratic Form (Part 2)

equation reducible to quadratic equation

Presenting the integral value of the equation in mathematics can be referred to as the positive sign of the quadratic equation. So, check that the equation can be reduced to quadratic form, then apply a simple substitution to convert it to a quadratic equation. Different situations in the practical world use cases often desire to use the quadratic formula. The quadratic equation in standard form is essential when using the quadratic formula to solve it. In most circumstances, all that is required to determine whether it is reducible to quadratic form is to check that one of the exponents is twice the other. It is probably reducible to quadratic form if it is twice the other. Summary This article studied the definition and standard form of a quadratic equation.

Next