Use of complex numbers in electrical engineering. Why are imaginary numbers used in electrical engineering 2022-12-09

Use of complex numbers in electrical engineering Rating: 9,6/10 1633 reviews

Complex numbers are a mathematical concept that have found numerous applications in electrical engineering. They consist of a real part and an imaginary part, and can be represented in the form a + bi, where a is the real part and bi is the imaginary part. The imaginary unit, denoted by "i", is defined as the square root of -1.

One of the main uses of complex numbers in electrical engineering is in the analysis and design of electrical circuits. Complex numbers are particularly useful in this context because they allow engineers to represent both the magnitude and phase of a quantity, such as an electrical current or voltage.

For example, consider an electrical circuit that contains an inductor, which is a passive electrical component that stores energy in the form of a magnetic field. The current through an inductor is not in phase with the voltage across it, meaning that the phase angle between the current and voltage is not zero. By representing the current and voltage as complex numbers, it is possible to easily calculate the phase angle between them, which is important for understanding the behavior of the circuit.

Another important use of complex numbers in electrical engineering is in the analysis of alternating current (AC) systems. AC systems are commonly used to distribute electrical power over long distances, and the voltage and current in these systems oscillate at a specific frequency. By representing the voltage and current as complex numbers, it is possible to analyze the phase relationship between them, as well as the power being transmitted in the system.

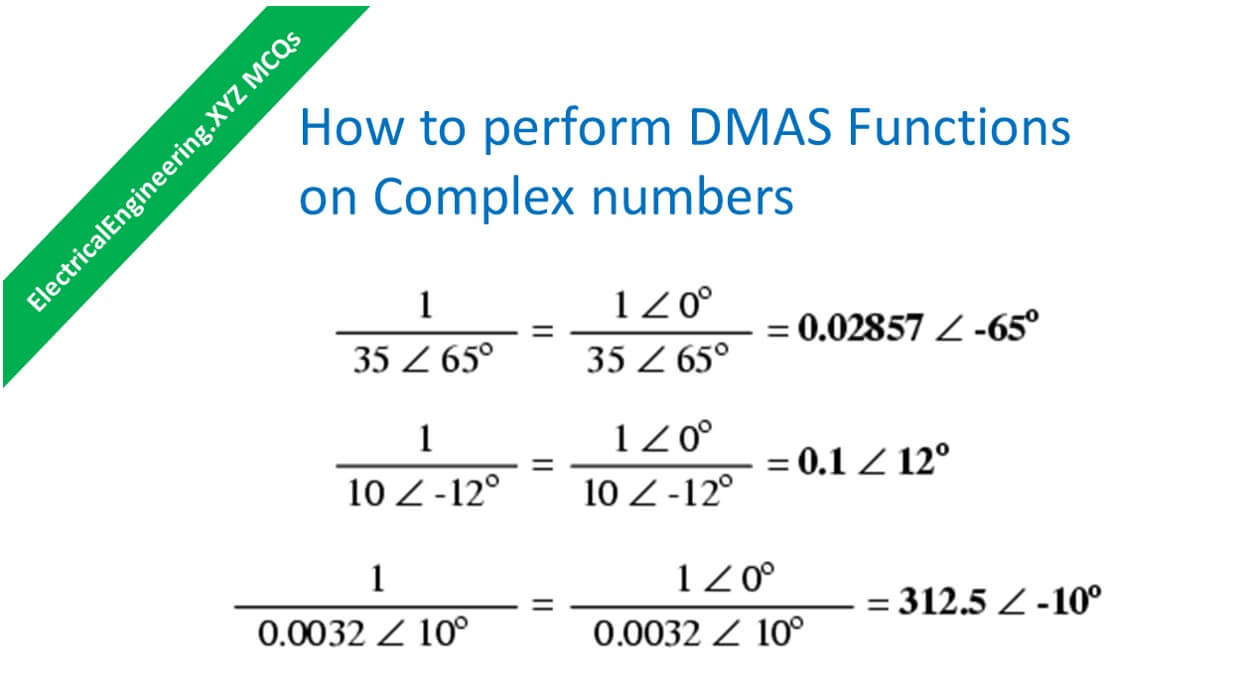
Complex numbers are also used in electrical engineering to represent the impedance of electrical components, such as resistors, capacitors, and inductors. Impedance is a measure of the opposition to the flow of electrical current in a circuit, and it is complex-valued because it includes both resistance (a real quantity) and reactance (an imaginary quantity). By representing impedance as a complex number, it is possible to easily calculate the total impedance of a circuit, which is important for understanding the behavior of the circuit.

In summary, complex numbers are an essential tool in electrical engineering, with numerous applications in the analysis and design of electrical circuits, the analysis of AC systems, and the representation of impedance. Their ability to represent both magnitude and phase makes them a powerful tool for understanding and predicting the behavior of electrical systems.

Application of Complex Numbers (Electrical Engineering)

use of complex numbers in electrical engineering

In using the arc-tangent formula to find the angle, we must take into account the quadrant in which the complex number lies. Hello I hope you are all doing well, in these difficult times. The main reason for this is they make the whole topic of analyzing and understanding alternating signals much easier. In applied fields, complex numbers are often used to compute certain real-valued improper integrals, by means of complex-valued functions. It is not impossible to do that without complex numbers but it is more convenient. If there are multiple frequencies, you can study the network at each frequency, and add the results super impose them. What is a complex number with two parts? I just use complex numbers every day without thinking about it.

Next

How complex numbers are used in electrical engineering problems?

use of complex numbers in electrical engineering

A complex number is a single mathematical quantity able to express these two dimensions of amplitude and phase shift at once. The conventional number system can be thought of as a straight line between minus infinity and plus infinity. The Laplace transform lets you conveniently move between the two, but even if you didn't have that you could still purely model and analyze the circuit in the time domain and then separately in the frequency domain. Recticing Software engineering in 21st century This technological manual explores how software engineering principles can be used in tandem with software development tools to produce economical and reliable software that is faster and more accurate. To divide, the radius equals the ratio of the radii and the angle the difference of the angles.

Next

Complex Numbers & Phasors in Polar and Rectangular Form

use of complex numbers in electrical engineering

The properties of the exponential make calculating the product and ratio of two complex numbers much simpler when the numbers are expressed in polar form. Curl your fingers around the base of your thumb. This third method is called the Exponential Form. As I learned about EE basics, I've learnt that complex numbers are VERY useful in EE and complex analysis is a must for students of EE. Do electricians use complex numbers? Negative numbers such as -3 and -5 are meaningless when measuring the mass of an object, but essential when keeping track of monetary debits and credits. How complex numbers are used in electronics? We have also seen that Complex Numbers can be presented in rectangular, polar or exponential form with the conversion between each complex number algebra form including addition, subtracting, multiplication and division.

Next

Why complex numbers are used/introduced in electricity

use of complex numbers in electrical engineering

The way to think about it is that GL contains matrices that are linear transformations. Thanks a lot, Sara While I agree with most of the comments already made, I would like to add, or emphasize, that complex numbers were introduced by Steinmetz in his theory of AC circuits because they can convert complicated problems into simpler, algebraic ones. Complex numbers are isomorphic to 2D vector algebra, except for complex number multiplication. However this is a very complicated topic and you need to read a couple of books to grasp it fully. Signal analysis Complex numbers are used insignal analysis and other fields for a convenient description for periodically varying signals.

Next

Why do we use complex numbers in electrical engineering?

use of complex numbers in electrical engineering

This allows us to analyse the AC Circuits involving Capacitors and Inductors easily without needing to use calculus. The Exponential Form uses the trigonometric functions of both the sine sin and the cosine cos values of a right angled triangle to define the complex exponential as a rotating point in the complex plane. It is particularly helpful to use complex numbers to model periodic phenomena, especially to operate with phase differences. Capacitor - a lot more complicated, but just picture as two metal plates wrapped in a protective cylinder. In order to accomplish this task, scalar numbers were abandoned andcomplex numberswere used to express the two dimensions of frequency and phase shift at one time. Why do engineers use complex numbers? Phasor notation is the process of constructing a single complex number that has the amplitude and the phase angle of the given sinusoidal waveform.

Next

2.1: Complex Numbers

use of complex numbers in electrical engineering

Many things in EE, as well as mechanics, and physics in general, go round in a circle, simple harmonic motion. Transients are very important, and the complex notation only applies when the system is in a "steady state". Sometimes the word phasor is used in electrical applications where the angle of the vector represents phase shift between waveforms. Is complex analysis useful for engineering? How do they apply to circuits? The treatment of resistors, capacitors, and inductors can then be unified by introducing imaginary, frequency-dependent resistances for the latter two and combining all three in a single complex number called the impedance. The quantity θ is the complex number's angle.

Next

Application of complex number in engineering

use of complex numbers in electrical engineering

Power, electronics, electromagnetics, semiconductors, software engineering, embedded systems - all topics relevant to this field, professional or academic. An imaginary number can't be numerically added to a real number; rather, this notation for a complex number represents vector addition, but it provides a convenient notation when we perform arithmetic manipulations. I have done some research concerning impedances and understand how they are written in complex form, however I am still confused why complex numbers are necessary in this field over regular numbers. OR if we had an understanding of which frequency we were working with beforehand, I could tell you the amplitude and time difference or phase, which is frequency normalized , and with just those two numbers, you could perfectly reconstruct what I'm thinking of. When the original complex numbers are in Cartesian form, it's usually worth translating into polar form, then performing the multiplication or division especially in the case of the latter.

Next

Basics of Complex Numbers for Electrical Engineers

use of complex numbers in electrical engineering

And even if that's the dominant power system we have, saying electricity is mostly about cyclical things can be erroneous. Good luck on your interview! Residue theorem The residue theorem in complex analysisis a powerful tool to evaluate path integrals of meromorphic functions over closed curves and can often be used to compute real integrals as well. Understanding information and power system designs and developing new systems all hinge on using complex numbers. In general they are used when some quantity has a phase as well as a magnitude. Physicists and electrical engineers may routinely work with complex numbers. In electrical engineering, the Fourier transform is used to analyze varying voltages and currents.


Next